Modeling manufacturing by precipitation - A need for catalyst supports

In chemical conversion processes implementing heterogeneous catalysis, the active phase, which accelerates molecular conversions, is often deposited on a porous support. Most of the time, this support has a large internal surface area, making it possible to host a large number of active sites within a small volume. Very often, the porous support is alumina, which needs to have adequate mechanical and thermal resistances and enhance mass and heat transfer. These properties are highly dependent on the texture of the support, which itself stems from its production process.

For alumina supports, this process hinges around a coagulation phenomenon: the assembly of primary boehmite crystallites^a (between 1 and 5 nm) forms highly stable aggregates (between 10 and 50 nm) which then form agglomerates (between 100 nm and a few tens of μm) [1]. In practice, the production process begins with a boehmite powder mixed with an acid, in the form of a paste, in order to disperse the grains into small aggregate particles. Subsequently, a base is added to trigger coagulation and form agglomerates. This extremely chaotic agglomeration phenomenon involves an assembly on several size scales, from tens of nanometers to several microns. Its structure determines the final properties of the support.

Better controlling the production of alumina supports requires modeling of their final structure as a function of the physicochemical parameters involved. To this end, a PhD thesis^b focused on the dynamics of the agglomeration in static conditions (i.e., in the absence of hydrodynamic forces).

To quantify the impact of pH and ionic strength, as well as boehmite concentration, on agglomeration kinetics, three experimental techniques were used: dynamic light scattering (DLS), small-angle x-ray scattering (SAXS) and scanning transmission electron microscopy (STEM). This multi-technique characterization approach made it possible to highlight the structural properties on various scales. This information was exploited via a population balance equation, using a Brownian dynamics model^c. The population balance results were then used to define the parameters for a morphological agglomeration model, capable of simulating the final porous structure of the solid alumina. A digital twin of the 3D morphology of an agglomerated boehmite grain within a colloidal suspension was thus created. The latter takes into account structural parameters such as size distribution, fractal dimension^d and assembly order.

The microstructure of the final solid was simulated in several steps using two successive physical models: a Lagrangian Brownian dynamics model and a population balance model.

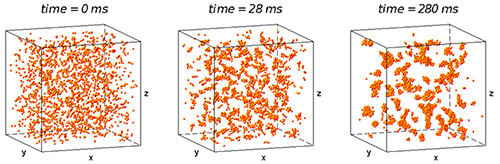
The first step consisted in building a morphological model of crystallite aggregation (figure 1). Various sticking probabilities were allocated to the concave and non-concave points of an aggregate and associated with physicochemical parameters. The first model provided information about the relationship between the fractal dimension and agglomerate mass, a property confirmed by the results of the SAXS analysis [2].

This relationship was then exploited in a population balance model to enable a realistic simulation of a large-sized colloidal system. The experimental DLS results were used to adjust the model’s parameters.

Lastly, the size distribution and fractal dimension obtained from the population balance were used to define the parameters for the global morphological model and build a 3D aggregate agglomerate.

The morphological model developed integrates the operating conditions required to generate a suitable 3D structure and can be further improved. For example, non-spherical particles could be included in the Brownian model. Moreover, by directly considering boehmite crystallites as primary particles rather than their aggregates, it will eventually be possible to calculate the textural properties of the alumina support, such as pore volume, specific surface area, pore size distribution and tortuosity. Another possible extension to get closer to industrial operating conditions is to consider shear at higher solid concentrations, inducing forced agglomeration and fracture phenomena.
 

^a- Common name for aluminum hydroxide oxide, a natural hydrated aluminum oxide and constituent of bauxites.
^b- PhD thesis by G. Ferri: “Identification and study of relevant descriptors of the solid during the synthesis of boehmite”, Paris-Saclay University, 2021.
^c- Simulation method used to describe the dynamics of particles interacting in a highly damped environment.
^d- This dimension characterizes the compactness of the structure of a particle assembly. Its value tends to 1, 2 or 3 respectively for linear, planar and spherical clusters. Open structures have a low fractal dimension while compact structures have a high fractal dimension.
     

References:

1. G. Ferri, S. Humbert, M. Digne, J-M. Schweitzer, M. Moreaud, Simulation of Large Aggregate Particles System with a New Morphological Model, Image Analysis & Stereology, 2021, vol. 40, pp 71-84. 
   >> https://www.ias-iss.org/ojs/IAS/article/view/2488
     

2. G. Ferri, S. Humbert, J-M. Schweitzer, M. Digne, V. Lefebvre, M. Moreaud, Mass fractal dimension from 2D microscopy images via an aggregation model with variable compactness, Journal of Microscopy, 2022, vol. 286, n° 1, pp 31-41.
   >> DOI: https://doi.org/10.1111/jmi.13088
       

Scientific contact: jan.verstraete@ifpen.fr

>> ISSUE 55 OF SCIENCE@IFPEN